Need higher SAT Math scores? Check out the best SAT math prep book ever written!
How to Solve a System of Equations
On both the SAT and ACT, you can bet that you’ll be required to solve a system of equations at least once. Probably more than once, if we’re being honest. Yet many students struggle with this task, which is actually very straightforward. In order to master it, we’re going to assume you have basic algebra skills. First, we’ll cover what a system of equations is. Next, we’ll explain what it means when a system has a solution (or solutions). Then, we’ll learn the basic principle to keep in mind when solving a system of equations. After that, we’ll show you three useful ways to solve a system of equations and offer some final tips. Ready? Let’s go!
What is a System of Equations?
Simply put, a system of equations is more than one equation! Think of your graphing calculator’s ability to graph more than one function at once–that’s what a system of equations is, simply more than one equation considered at a time. It might be two lines, two parabolas, a parabola and a line–whatever.
What Does It Mean When a System of Equations Has “Solutions”?
When you solve for the solution (or solutions) of a system of equations, you’re finding the point (or points) at which the functions INTERSECT. These points will work in ALL equations in the system. Two lines that are parallel have NO solution. Two functions that are the SAME (perhaps just in different forms) have an INFINITE number of solutions. Two lines that intersect once have ONE solution. A line might intersect with a parabola twice to give TWO solutions, and so on. So, when you’re solving a system of equations, you’re finding the point or points on the X-Y coordinate plane at which the functions INTERSECT.
What’s the Basic Principle for Solving a System of Equations?
Honestly, this principle is EXTREMELY important for students who struggle with algebra to keep in mind. Algebra’s pretty easy when you’ve got one variable, isn’t it? X + 5 = 9 doesn’t pose much of a challenge, nor does 3x+9 = 27.
But what if you have MORE than one variable, such as X and Y? Or X, Y, and Z? Then algebra becomes a little trickier–it might almost seem impossible! It isn’t, however.
The secret principle to solving systems of equations (and for doing algebra with more than one variable) is the following series of questions:
- Algebra is easy when there’s one variable.
- What variable do I want?
- What do I want to get rid of right now?
- How do I eliminate it?
Basically, this series of questions will give you a mission when you’ve got a system of equations. It will help you solve the system via substitution or elimination. These are basically the same thing–you’re solving for one variable and plugging the result into the other equation, but with elimination, you’re manipulating one equation a little more. We’ll talk about that soon. For now, let’s look at substitution.
Using SUBSTITUTION to Solve a System of Equations
Remember that algebra’s easy when there’s just one variable. But in a system of equations, you’ve got a couple x’s and a couple y’s. No good. The solution? Simply SOLVE FOR ONE VARIABLE and PLUG THE RESULT INTO THE OTHER EQUATION to find the other variable. Let’s use a sample SAT problem to see this technique in action.
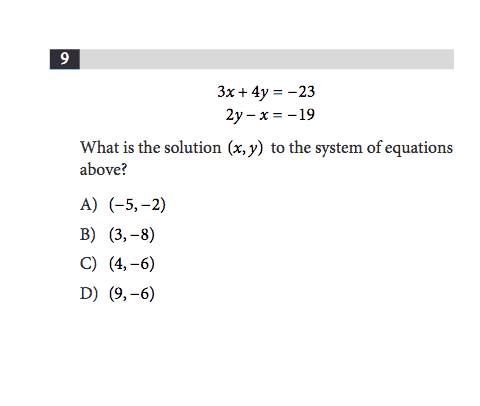
If your first instinct is to start plugging those answers choices into the equations given, resist the impulse! That’s totally one way to solve the problem, but what happens when you DON’T have multiple choice answers to go by? Let’s solve this system via substitution.
First, we’re going to solve for one variable in one equation. Then, we’re going to plug that answer into the other equation to find the second variable. Take a look at the two equations. Which one seems to be the easiest one to solve for a variable? I like the second equation, and I’m going to solve for x. Follow along as I use algebra to solve for x.
2y - x = -19
2y = -19 + x
2y + 19 = x
Hooray! Now I know that x = 2y + 19. Time to plug that result into the other equation. Again, watch what happens:
3x + 4y = -23
3(2y + 19) + 4y = -23
6y + 57 + 4y = -23
10y + 57 = -23
10y = -80
y = -8
Yes! Now I know the value of y is -8. How do I get the value of x? It’s simple. I’ll plug my value of y into either of the equations. Let’s plug it into the original equation.
2y - x = -19
2(-8) - x = -19
-16 - x = -19
-16 = -19 + x
3 = x
Awesome. I’ve found the value of x is 3, and I know from my earlier calculations that the value of y is -8. That makes the solution to this problem (3, -8) or answer choice B! The point these lines intersect is (3, -8).
Using Elimination to Solve a System of Equations
Now that we’ve learned how to use substitution, let’s take a look at another way to solve a system of equations: elimination. Sometimes this method can be MUCH faster than substitution, so it’s worth pondering which method might be best before you begin working.
Back to our original problem…

This time, our goal is to eliminate a variable by combining the two equations. You see how in the top equation we have 4y and in the bottom equation we have 2y? If we wanted to get rid of y, we need to make 2y into 4y and subtract the equations or make 2y into -4y and add the equations. If we wanted to get rid of x, we would need to turn the -x of the bottom equation into -3x or 3x to add or subtract the equations. It doesn’t matter which variable you choose to eliminate! Let’s choose x.
In order to add the equations together, I need to turn the -x in the second equation into -3x. How do I do that? Easy. I’ll multiply the bottom equation by 3! In algebra, you can do anything you like to an equation as long as you do the same thing to BOTH sides. So let’s multiply the bottom equation by 3!
2y - x = -19
becomes
6y - 3x = -57
Now we can add our two equations.
3x + 4y = -23
+
6y - 3x = -57
=
10y = -80
Look familiar? That’s because substitution and elimination are two ways to go about the same thing.
Of course, y = -8. Plugging that into either equation gives us x = 3, but I’m sure you guessed that!
Solving a System by Plugging in Answers
Of course, you can always solve a system of equations by plugging in answer choices. Or, if you want, you could graph both of the equations and find their point of intersection. We will ALWAYS prefer algebra, however, because it’s so important to learn and practice!
For more practice on SAT-style Systems of Equations, you need SAT Math Mastery Vol.2. It has a massive lesson on every type of Systems of Equations question on the SAT math test, and it’s filled with SAT math practice and strategies. I also recommend getting Volume 1 for all the essential SAT Algebra concepts. Order the textbook on Amazon or learn more about it heres!
That’s it! For more algebra tips, as well as a ton of SAT and ACT prep advice, check out the rest of our blog. Looking for 1-on-1 ACT or SAT prep tutoring? Want to join an SAT or ACT group class? Contact us today!